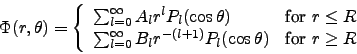Next: About this document ...
Up: tut4
Previous: tut4
- Griffiths (3.17(b))
- Find the potential inside and outside a sphere
shell that carries a uniform surface charge
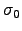 , using results of Ex. 3.9
, using results of Ex. 3.9
We know the potential inside and out side must have a form
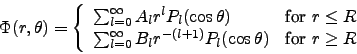 |
(1) |
By continuity of the potential,
The normal component of the electric field is discontinuous by
 ,
,
Thus,
We can find 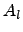 ,
,
Since 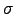 is constant,
is constant,
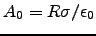 , and is 0 for all other
, and is 0 for all other 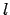 .
Then the potential is given by
.
Then the potential is given by
 |
(2) |
- Griffiths (3.18)
- The potential at the surface of a sphere (radius
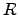 ) is
given by
) is
given by
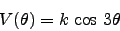 |
(3) |
where  is a constant. Find the potential inside and outside the sphere, as well as
the charge density
is a constant. Find the potential inside and outside the sphere, as well as
the charge density
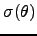 on the sphere. (Assume that there is no charge
inside or outside the sphere.)
on the sphere. (Assume that there is no charge
inside or outside the sphere.)
First, notice that
![$V(\theta)=k\cos\,3\theta=(k/5)[8 P_3(\cos\theta)-3 P_1(\cos\theta)]$](img17.png) .
Potential is given by Eq. 1. One can find
.
Potential is given by Eq. 1. One can find 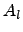 by comparing
by comparing
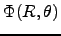 with
with  .
.
The charge density
- Griffiths (3.21)
- In Prob. 2.25 you found the potential on the axis of a
uniformly charged disk:
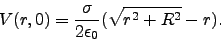 |
(4) |
(a) Use this, together with the fact that  , to evaluate the first three
terms in the expansion (3.72) for the potential of the disk at points off
the axis, assuming
, to evaluate the first three
terms in the expansion (3.72) for the potential of the disk at points off
the axis, assuming 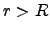 .
(b) Find the potential for
.
(b) Find the potential for 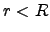 by the same method, using (3.66).[Note: You must
break the interior region up into two hemispheres, above and below the disk. Do
not assume the coefficients
by the same method, using (3.66).[Note: You must
break the interior region up into two hemispheres, above and below the disk. Do
not assume the coefficients 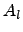 are the same in both hemispheres.
are the same in both hemispheres.
For 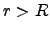 , on the Z-axis,
, on the Z-axis,
![\begin{displaymath}
V(r,0)= \frac{\sigma}{2\epsilon_0}(\sqrt{r^2+R^2}-r)
=\fr...
..._0} \left[
\frac{R^2}{2r} - \frac{R^4}{8r^3}+\cdots
\right]
\end{displaymath}](img29.png) |
(5) |
The Eq. 1 must match with this expression at 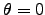 . Thus:
. Thus:
![\begin{displaymath}
V(r,\theta)= \frac{\sigma}{2\epsilon_0}(\sqrt{r^2+R^2}-r)
...
...\cos\theta) - \frac{R^4}{8r^3}P_2(\cos\theta)+\cdots
\right]
\end{displaymath}](img31.png) |
(6) |
For 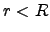 , the volume is not free of charge. Thus must be divided into two charge free
regions. The expansion in terms of the Legendre polynomials will be different in different
regions.
In north hemisphere,
, the volume is not free of charge. Thus must be divided into two charge free
regions. The expansion in terms of the Legendre polynomials will be different in different
regions.
In north hemisphere,
![\begin{displaymath}
V(r,0)= \frac{\sigma}{2\epsilon_0}(\sqrt{r^2+R^2}-r)
=\fr...
...t[
R - r + \frac{r^2}{2R} - \frac{r^4}{8R^3}+\cdots
\right]
\end{displaymath}](img32.png) |
(7) |
Thus
![\begin{displaymath}
V(r,\theta)= \frac{\sigma}{2\epsilon_0}(\sqrt{r^2+R^2}-r)
...
...os\theta)
- \frac{r^4}{8R^3}P_4(\cos\theta)+\cdots
\right]
\end{displaymath}](img33.png) |
(8) |
For southern hemisphere,
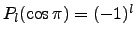 , hence
, hence
![\begin{displaymath}
V(r,\theta)= \frac{\sigma}{2\epsilon_0}(\sqrt{r^2+R^2}-r)
...
...os\theta)
- \frac{r^4}{8R^3}P_4(\cos\theta)+\cdots
\right]
\end{displaymath}](img33.png) |
(9) |
- Griffiths (3.37)
- A conducting sphere of radius
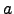 , at potential
, at potential 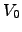 ,
is surrounded by a thin concentric spherical shell of radius
,
is surrounded by a thin concentric spherical shell of radius 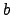 , over which someone
has glued a surface charge
, over which someone
has glued a surface charge
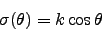 |
(10) |
where  is a constant, and
is a constant, and 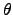 is a usual spherical coordinate.
is a usual spherical coordinate.
(a) Find potential in each region: (i) 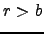 (ii)
(ii) 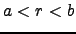 .
.
(b) Find the induced charge density
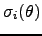 on the conductor.
on the conductor.
(c) What is the total charge of this system? Check that your answer is consistent
with the behaviour of 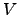 at large
at large 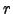 .
.
The form of potential is
 |
(11) |
Now for each 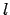 , we have to determine three constants
, we have to determine three constants
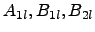 . We have three
conditions of potential: (i) At
. We have three
conditions of potential: (i) At  , potential is
, potential is 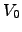 . (ii) At
. (ii) At 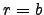 , potential must be
continuous. (iii) At
, potential must be
continuous. (iii) At 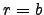 , normal component of electric field must be discontinuous by
, normal component of electric field must be discontinuous by
 .
.
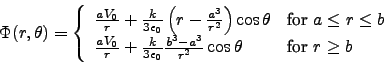 |
(12) |
The charge density on the conducting surface
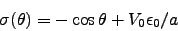 |
(13) |
- Jackson (3.1)
- Two concentric spheres have radii
 and each s divided
into two hemispheres by the same horizontal plane. The upper hemisphere of the inner sphere
and the lower hemispheres of the outer sphere are maintained at potential
and each s divided
into two hemispheres by the same horizontal plane. The upper hemisphere of the inner sphere
and the lower hemispheres of the outer sphere are maintained at potential 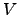 . The other
hemispheres are at zero potential.
. The other
hemispheres are at zero potential.
Determine the potential in the region 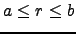 as a series of Legendre polynomials.
Include terms at least upto
as a series of Legendre polynomials.
Include terms at least upto 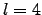 . Check your solution against known results in the limiting
cases
. Check your solution against known results in the limiting
cases
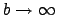 and
and
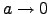 .
.
Begin with a general solution
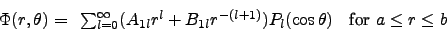 |
(14) |
Apply boundary conditions at both the surfaces:
- Jackson (3.2)
- A spherical surface of radius
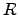 has charge uniformly distributed over
its surface with a density
has charge uniformly distributed over
its surface with a density 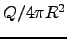 , except for a spherical cap at the north pole,
defined by a cone
, except for a spherical cap at the north pole,
defined by a cone
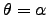 .
.
(a) Show that the potential inside the spherical surface can be expressed as
![\begin{displaymath}
\Phi = \frac{Q}{8\pi\epsilon_0}\sum_{l=0}^\infty\frac{1}{2l...
...alpha)-P_{l-1}(\cos\alpha)]\frac{r^l}{R^{l+1}}P_l(\cos\theta)
\end{displaymath}](img63.png) |
(17) |
where, for 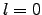 ,
,
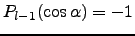 . What is the potential outside?
. What is the potential outside?
(b) What is the magnitude and the direction of the electric field at the origin?
(c) Discuss the limiting form of the potential(part a) and electric field (part b)
as the spherical cap becomes (i) too small, and (ii) so large that the area with the
charge on it becomes a very small cap at the south pole.
First we calculate potential at the points on the z axis.
Since,
![$\int_{-1}^{\cos\alpha}P_l(x)dx = [P_{l+1}(\cos\alpha)-P_{l-1}(\cos\alpha)]/(2l+1)$](img69.png) by recurrence relation. Thus,
by recurrence relation. Thus,
![\begin{displaymath}
\Phi(z) = \frac{Q}{8\pi\epsilon_0}\sum_{l=0}^\infty\frac{1}...
... [P_{l+1}(\cos\alpha)-P_{l-1}(\cos\alpha)]\frac{r^l}{R^{l+1}}
\end{displaymath}](img70.png) |
(20) |
The required result is immediate.
For part b,
 |
(21) |



Next: About this document ...
Up: tut4
Previous: tut4
Charudatt Kadolkar
2007-02-23
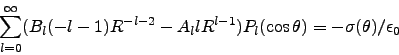
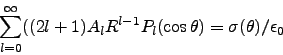

![\begin{displaymath}
\Phi(r,\theta)= \left\{
\begin{array}{ll}
\frac{k}{5}\lef...
...ht] & \mbox{for $r\ge R$\ }\\
\end{array} \right. \nonumber
\end{displaymath}](img20.png)
![$\displaystyle -\epsilon_0 \left[ \frac{\partial\Phi}{\partial r}(R_+) -
\frac{\partial\Phi}{\partial r}(R_-) \right]$](img23.png)
![$\displaystyle \epsilon_0 \frac{k}{5R}\left[ 56 P_3(\cos\theta)
- 9 P_1(\cos\theta) \right]$](img24.png)
![\begin{displaymath}
V(r,0)= \frac{\sigma}{2\epsilon_0}(\sqrt{r^2+R^2}-r)
=\fr...
..._0} \left[
\frac{R^2}{2r} - \frac{R^4}{8r^3}+\cdots
\right]
\end{displaymath}](img29.png)
![\begin{displaymath}
V(r,\theta)= \frac{\sigma}{2\epsilon_0}(\sqrt{r^2+R^2}-r)
...
...\cos\theta) - \frac{R^4}{8r^3}P_2(\cos\theta)+\cdots
\right]
\end{displaymath}](img31.png)
![\begin{displaymath}
V(r,0)= \frac{\sigma}{2\epsilon_0}(\sqrt{r^2+R^2}-r)
=\fr...
...t[
R - r + \frac{r^2}{2R} - \frac{r^4}{8R^3}+\cdots
\right]
\end{displaymath}](img32.png)
![\begin{displaymath}
V(r,\theta)= \frac{\sigma}{2\epsilon_0}(\sqrt{r^2+R^2}-r)
...
...os\theta)
- \frac{r^4}{8R^3}P_4(\cos\theta)+\cdots
\right]
\end{displaymath}](img33.png)
![\begin{displaymath}
V(r,\theta)= \frac{\sigma}{2\epsilon_0}(\sqrt{r^2+R^2}-r)
...
...os\theta)
- \frac{r^4}{8R^3}P_4(\cos\theta)+\cdots
\right]
\end{displaymath}](img33.png)

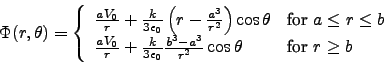
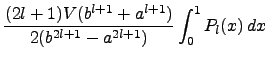
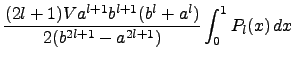
![\begin{displaymath}
\Phi = \frac{Q}{8\pi\epsilon_0}\sum_{l=0}^\infty\frac{1}{2l...
...alpha)-P_{l-1}(\cos\alpha)]\frac{r^l}{R^{l+1}}P_l(\cos\theta)
\end{displaymath}](img63.png)
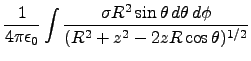
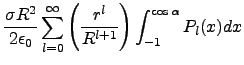
![\begin{displaymath}
\Phi(z) = \frac{Q}{8\pi\epsilon_0}\sum_{l=0}^\infty\frac{1}...
... [P_{l+1}(\cos\alpha)-P_{l-1}(\cos\alpha)]\frac{r^l}{R^{l+1}}
\end{displaymath}](img70.png)